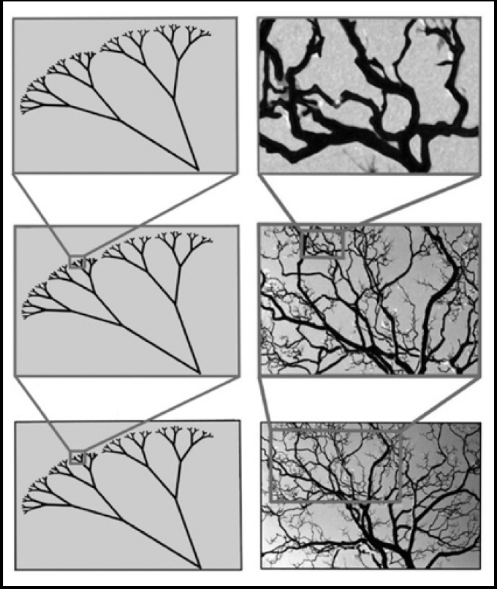
Fractals are commonly found within nature. They are self-similar structures, where one aspect of the fractile is identical to the rest. This allows it to be scaled up or down while fitting within itself. Within trees, fractalization is found in the way the branches are grown from each other, always yielding smaller and smaller versions of itself, starting with the trunk.

Fractalization and Structure
_______________________________
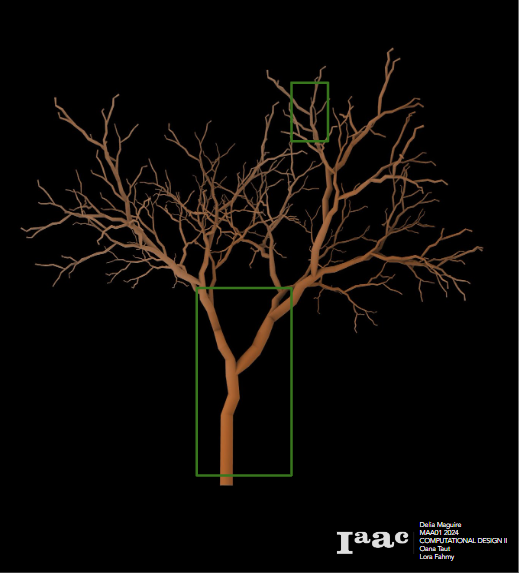
For trees to be able to stand, there are many factors that they must deal with. Gravity and wind and snow loads are those that match most closely within architecture. They do this in a number of ways, including: “reorienting structures to a reduced stress/strain position”[1].
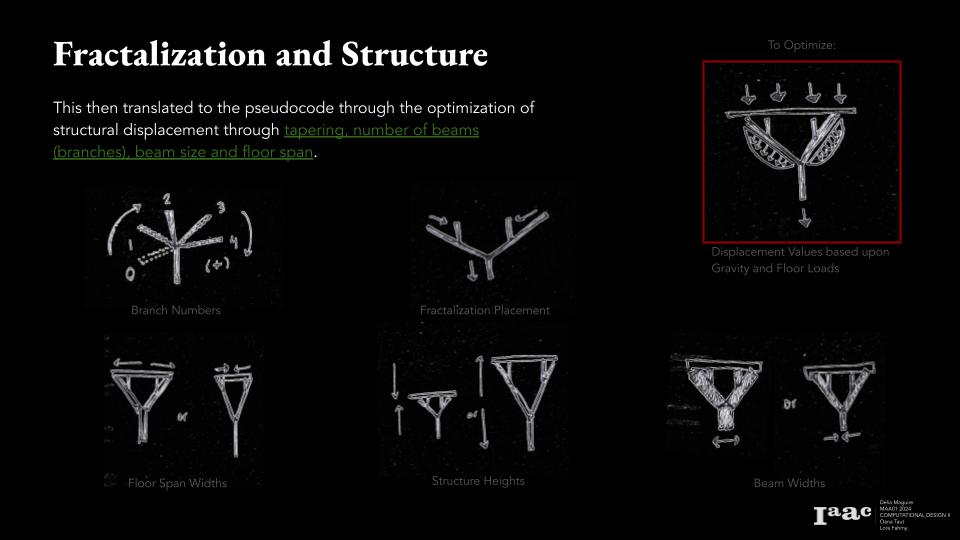
This happens through:
- The tapering or shrinking of fractal parts. This in term diminishes the stiffness of the parts helping to make parts less brittle.
- The crowning or flexibility that the tree gives to its branches.
- And the roots system, which act as a balance for the asymmetrical growth of the tree.
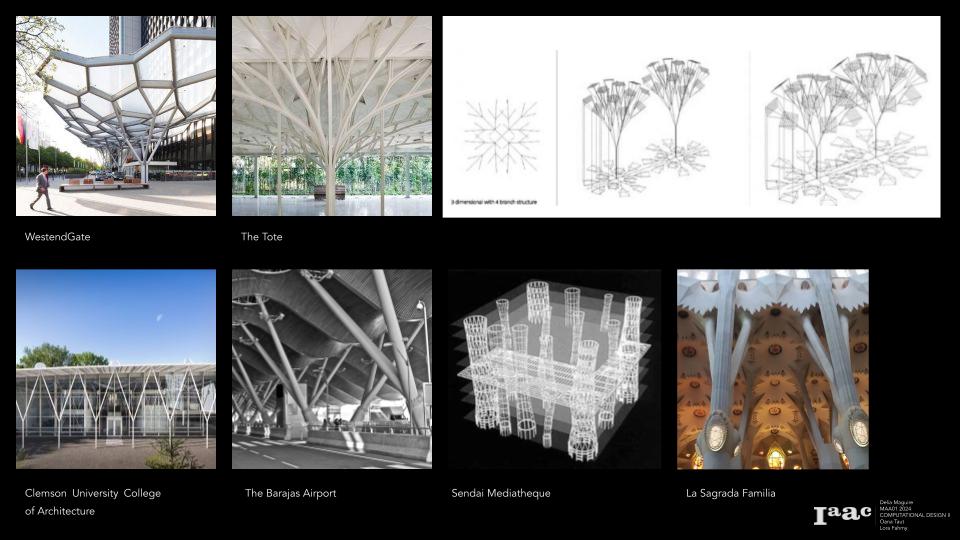
And can be seen throughout many examples:
Fractalization and Structure
_______________________________
Pseudocode
_______________________________
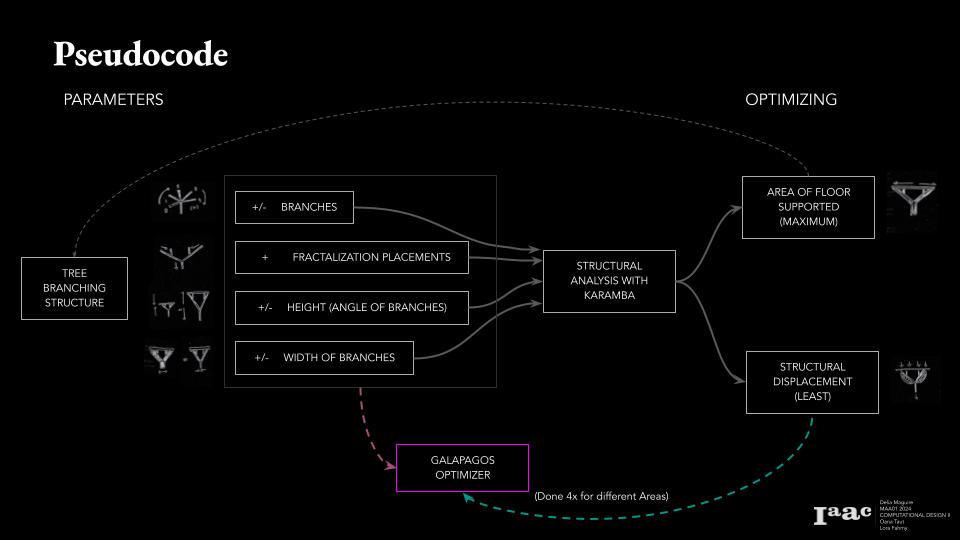
Optimization Results
_______________________________
Low Beam Displacement Catalogue
Indicating Structural Optimization:
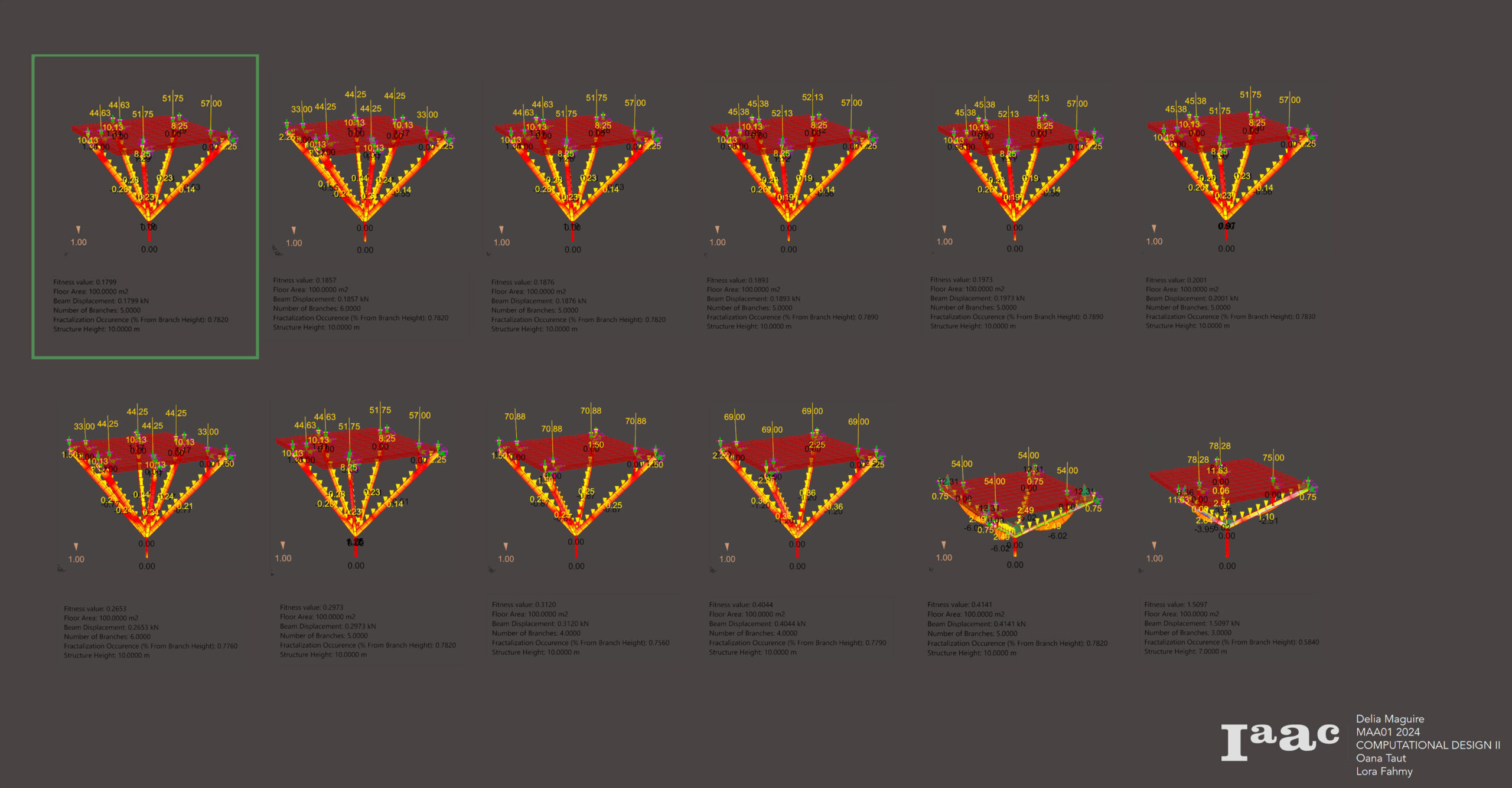
High Beam Displacement Catalogue
Indicating Structural Failure:
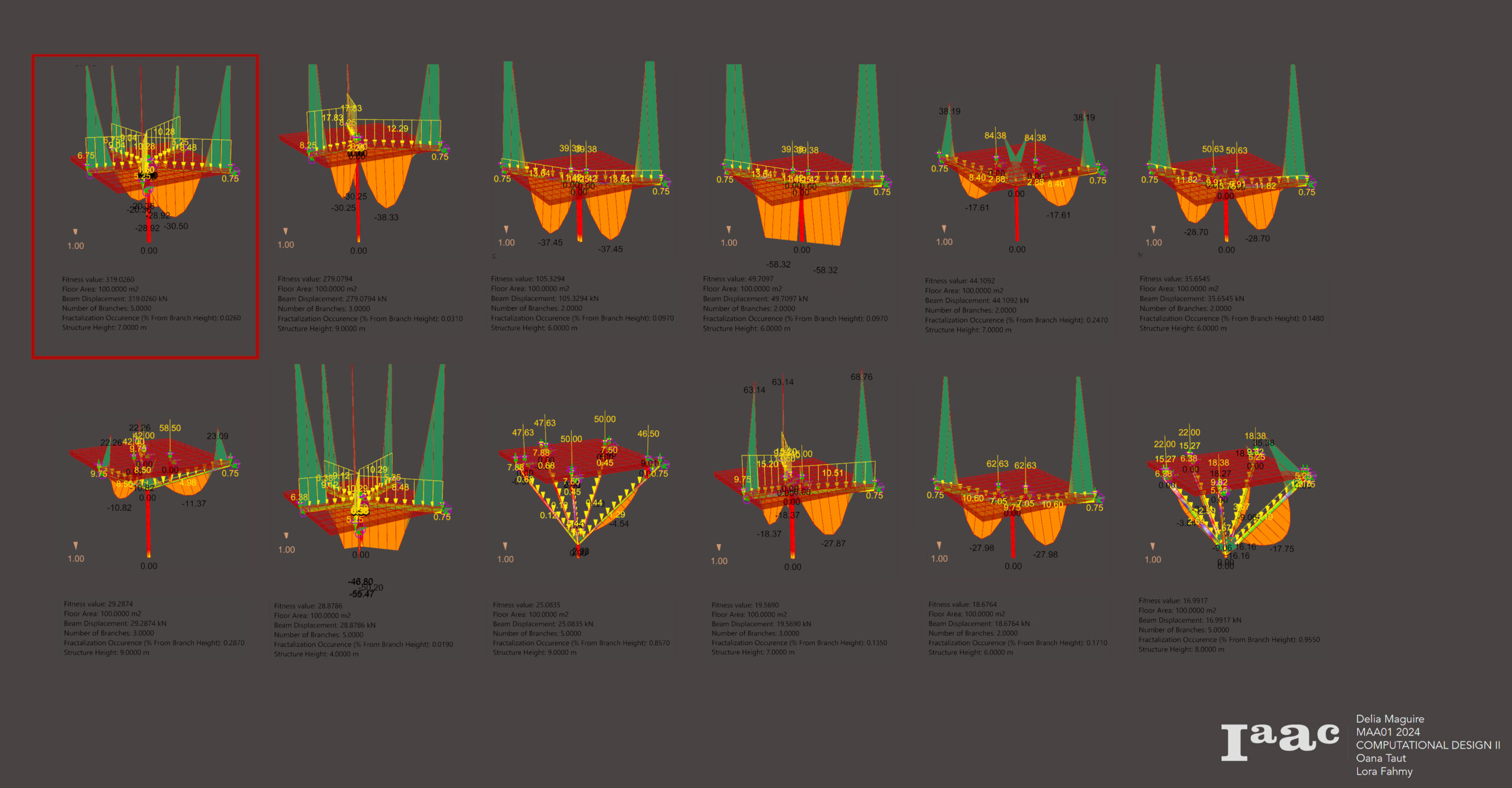
Sensitivity Analysis
_______________________________
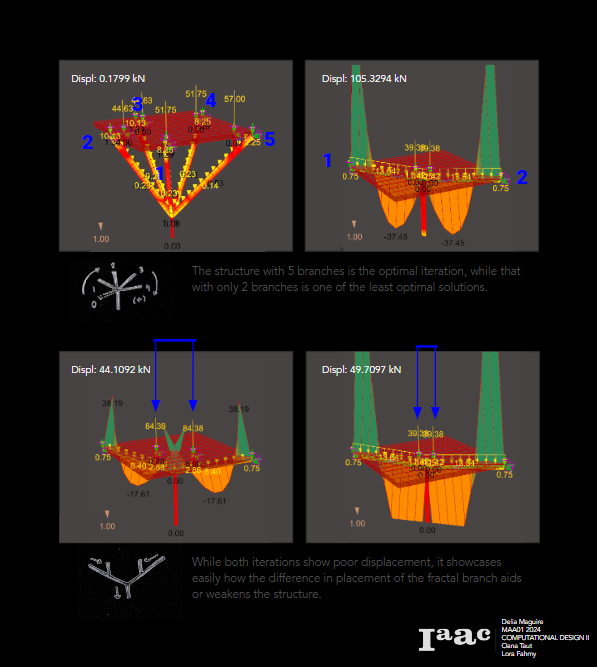
Through looking at the optimization iterations, it’s easy to distinguish that where there are more beams, there is less displacement within the structure. This can be concluded as due to the balancing of forces the beams are taking to the ground. The more there are, the less load per beam.
However, it is not just the number of beams, but their fractal placements that help or hurt the beams carrying load. Those that have fractals closer to the center have more displacement than those with fractal branches closer to the edges. This happens because the load is taken by the fractal branches in the center, they then take that load to the center of the branch beam which already has a load of its own. This causes stress in the middle of that beam, further from supports, thus causing more bending.
_______________________________
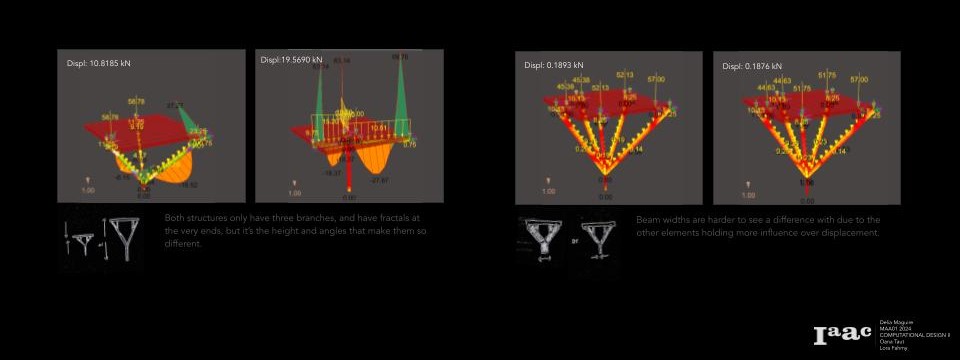
The heights and angles of the beams also give structural integrity. Those that are shorter and wider add more vertical load due to a larger span, making the displacement of the beams very high. While when the angle is more acute, the load is able to be carried much easier. This is because those with more horizontal span almost become cantilevered, which puts more strain on the branches than those that are more angled.
As the displacement values start to get smaller, and the optimization begins to steady, it really only begins to play with optimal beam widths. This is harder to conclude due to the small variations between the iterations, however matter in strength of the beam.
Lows and Highs
_______________________________
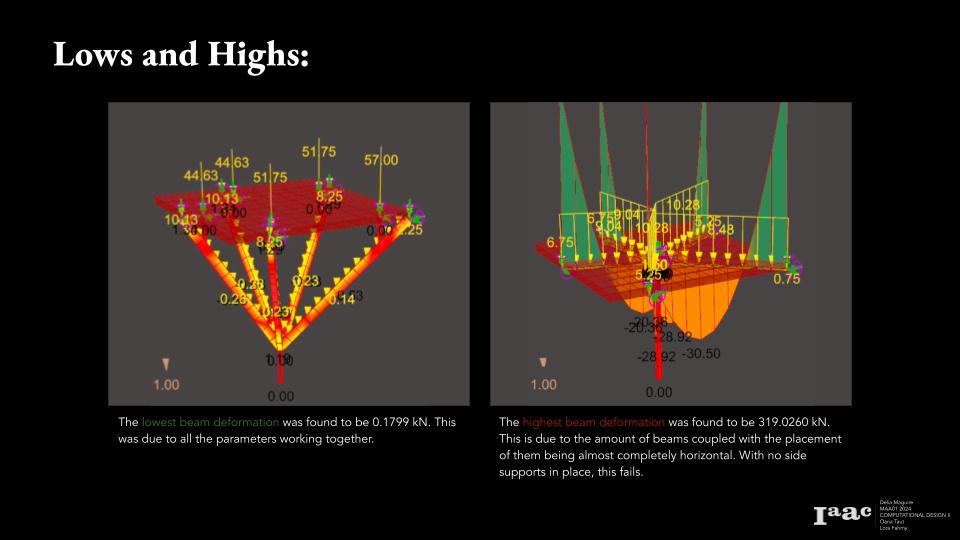
_______________________________
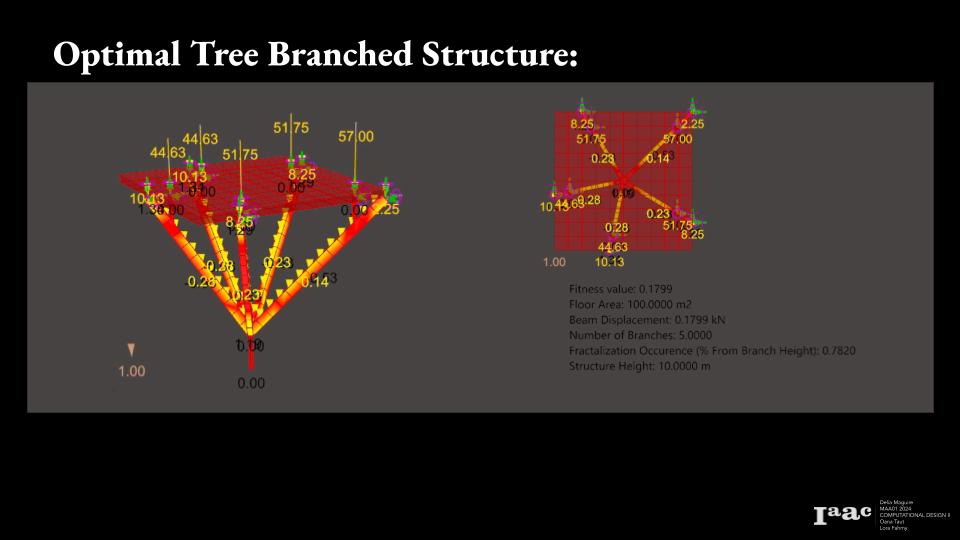
This beam structure is the most optimal since it allows a more equal distribution of load through the branch elements present. The fractalization optimization also allows less strain on the branch members. The angle and height of the structure also allows for an angle that gives the least cantilever effect, minimizing the effects of gravity. This is similar to how trees grow, the older branches become the more and more vertical and less horizontally spread they become [2].


