Design concept and system
Our topic was mathematical surfaces, so we started our project off that. We wanted to achieve a fluid and dynamic form, so we decided to combine strange attractors as a base for the building and a vector field as a facade system.

Early explorations – Strange Attractors
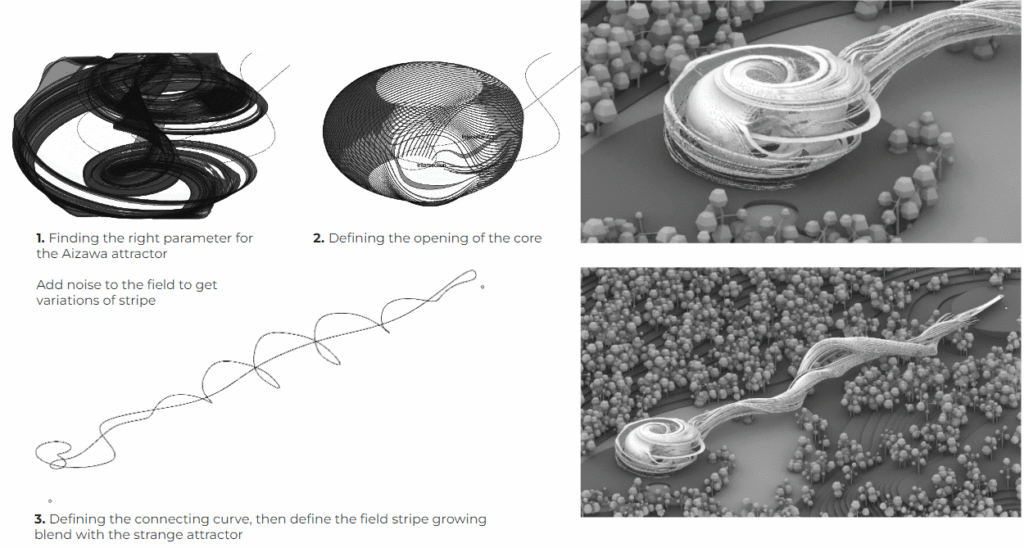
For the building form, we experimented with a few systems: the three-scroll unified chaotic system, the Rabinovich-Fabrikant curve, and the Aizawa attractor. After trying many iterations, we decided to expand on the Aizawa concept.

Computational design methodology
Our input mainly consisted of two points, which were the entrance and the destination. At the start point, we created a strange attractor and a field from it. From the field contour, we constructed a surface to enclose the building. Next, we connected the two points by creating a continuous field that let all the flow curves blend into part of the museum. Finally, we added details to the form, including additional structure, filling the function, and making a staircase inside the structure.
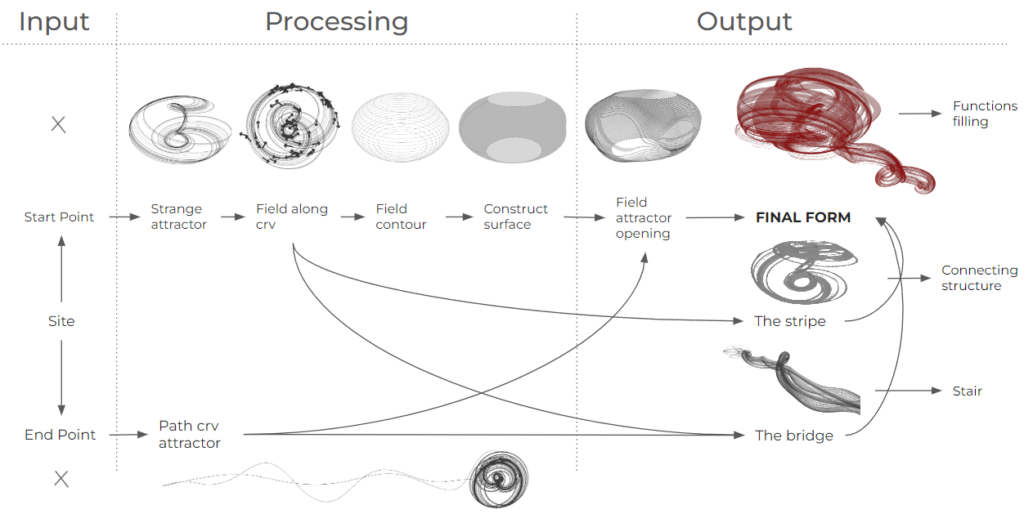
Form finding process
After modifying the attractor, we played with the opening. When the bridging curve intersects with the surface, it will trudge a Gaussian charge that moves the control point of the surface open. We bridged two points using a closed curve, which has one part already blended with the attractor core. The field curve was then flown to cover the bridge and become its structure.
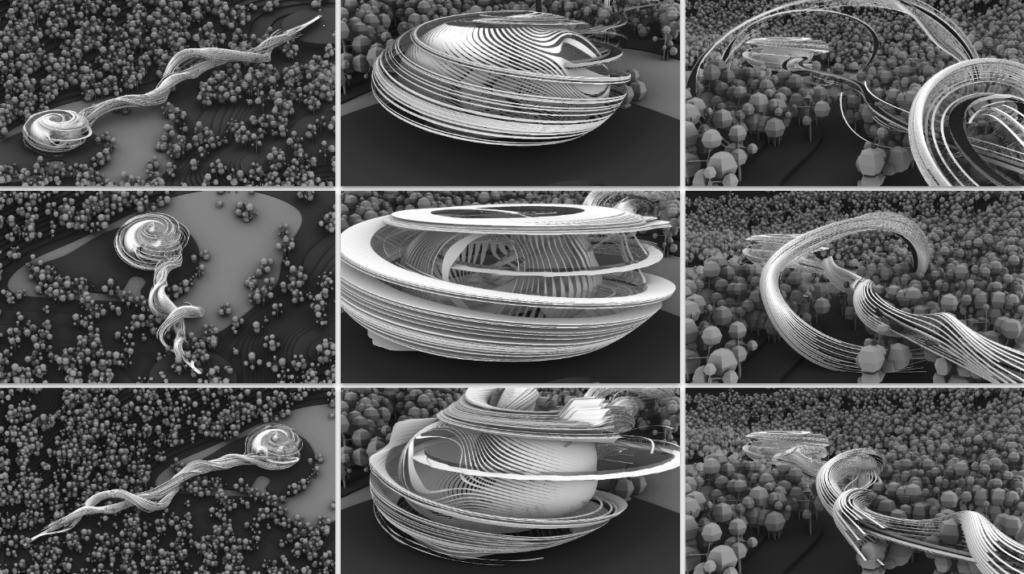
Form iterations
These were some variants that we created for the project. The continuous bridging field will always blend both the outside and the inside.
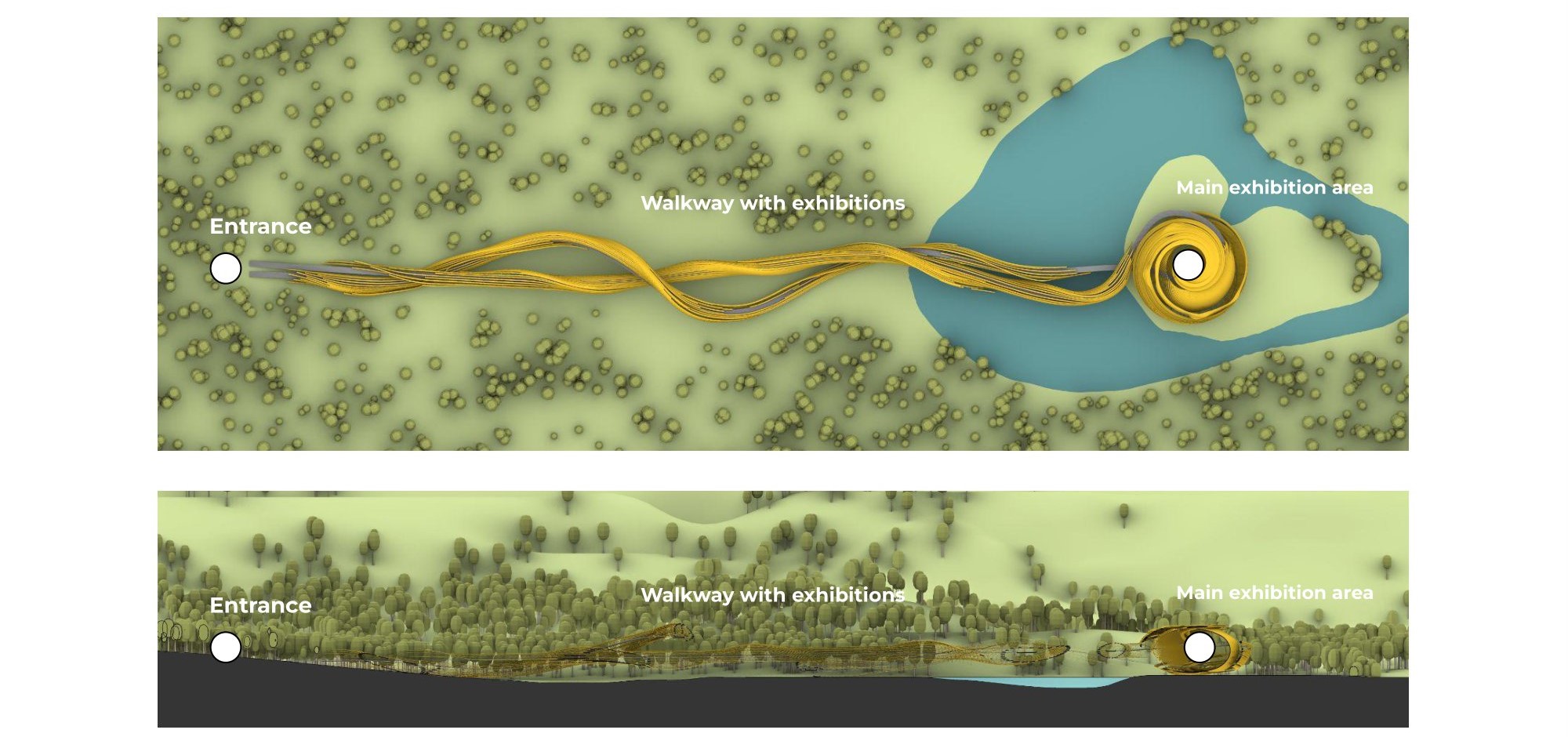
Layout and section
The layout of our structure was composed of three main parts: the entrance, a spiral walkway with smaller exhibitions, and the main building with larger exhibition areas.
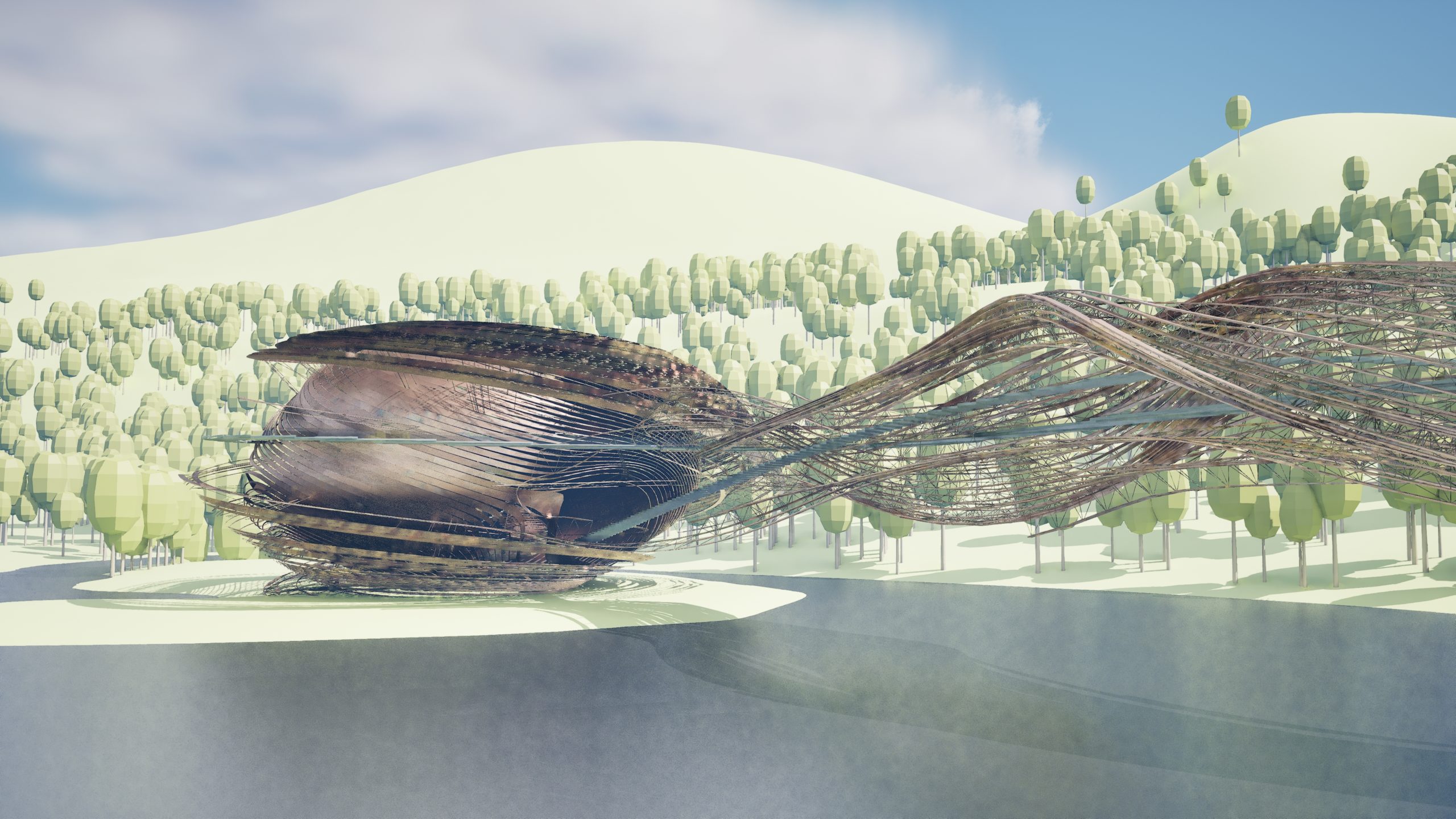
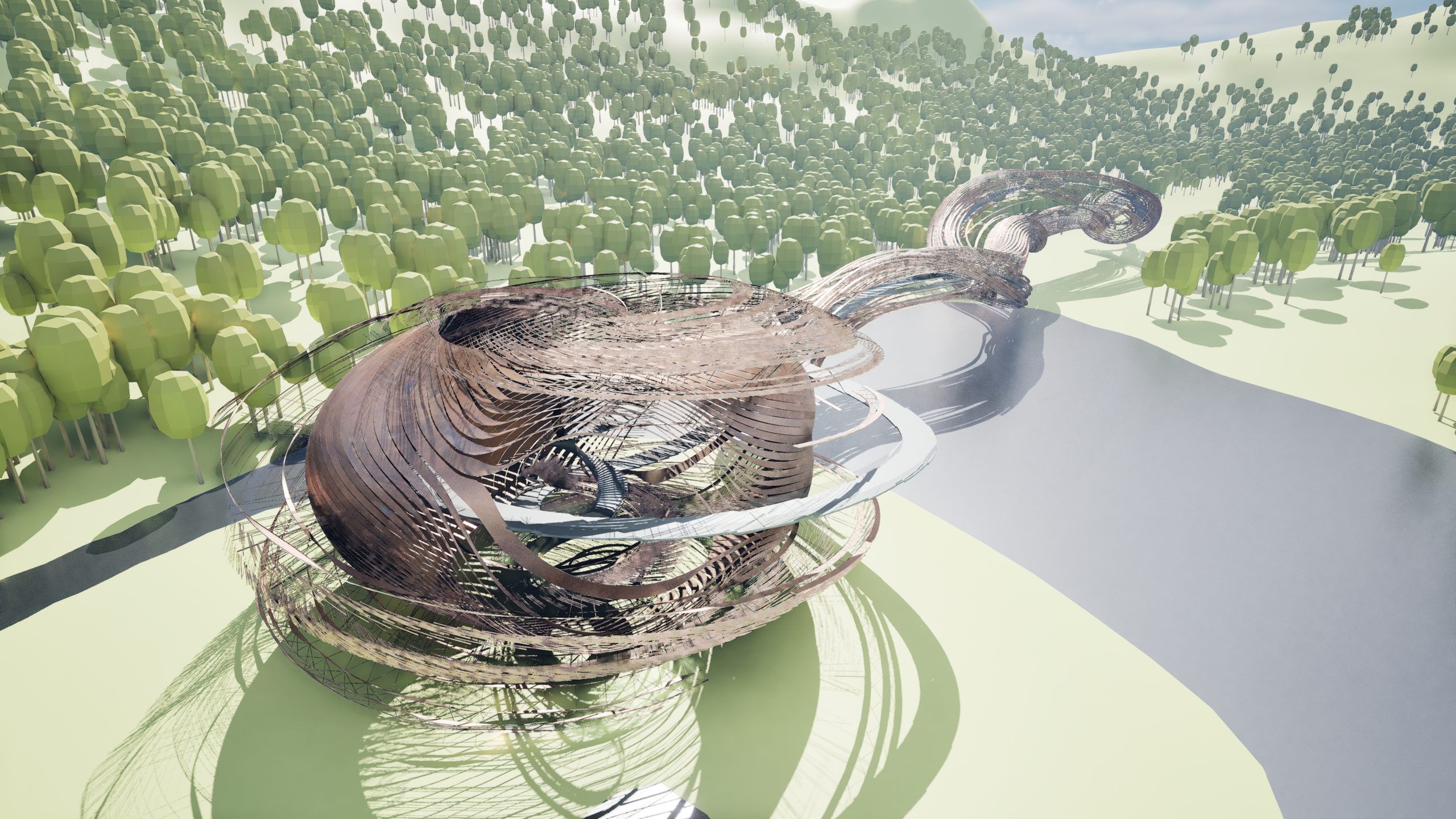
Renders
Aerial view
Human view